Mathematicians Excited About New 13-Sided Shape Called ‘the Hat’

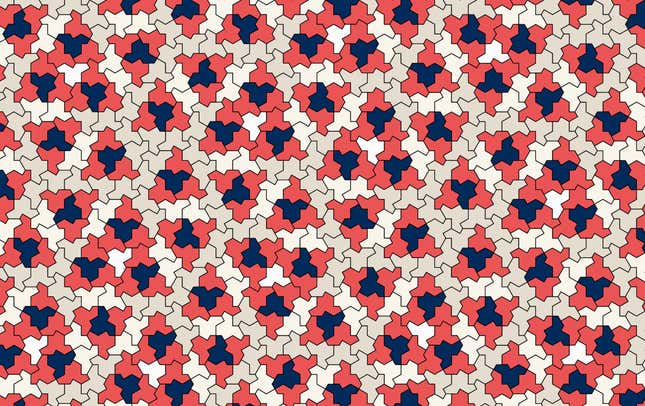
For some, tiles are rarely thought of unless it’s time for home renovations, but for mathematicians, they present plenty of conundrums—and a clever team has just cracked a particularly tricky one. Researchers identified a shape that was previously only theoretical: a 13-sided configuration called “the hat” that can tile a surface without repeating.

The hat is what’s known as an aperiodic monotile, which means that a single shape can tile a surface without any translational symmetry, or without its pattern ever repeating. The famous Penrose tilings are an example of aperiodic tiling, where the pattern is aperiodic but uses two different shapes.

The hat tiling only uses one shape, an “einstein,” which is German for “one stone,” making the pattern an aperiodic monotile. The 13-sided hat is a polykite shape, consisting of eight kites connected at their edges. The existence of an aperiodic monotile was purely theoretical until a research team led by mathematician David Smith and colleagues proved its existence in a preprint paper posted online this month.

“You’re literally looking for like a one in a million thing. You filter out the 999,999 of the boring ones, then you’ve got something that’s weird, and then that’s worth further exploration,” said co-author Chaim Goodman-Strauss, a mathematician at the National Museum of Mathematics, to New Scientist. “And then by hand you start examining them and try to understand them, and start to pull out the structure. That’s where a computer would be worthless as a human had to be involved in constructing a proof that a human could understand.”

G/O Media may get a commission

up to 70% off

Lenovo Spring Clearance

Deals on deals
Take up to 70% off at Lenovo’s Spring Clearance sale, including a wide range of laptops, monitors, and tablets. Take an extra 15% off with the promo code.

Advertisement

For mathematicians, the discovery appears to answer a long-standing question in the field of geometry. But for the rest of us, perhaps it represents a funky new option for bathroom tile.